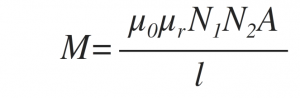As we know that, an inductor produces the induced emf as a result of the changing magnetic field around the coil of the inductor and when emf is induced in the same circuit this phenomenon is known as self-induction. However, if the emf is induced in the nearby coil within the same magnetic field, this emf is known as magnetically induced emf and the phenomenon is known as mutual induction. The symbol used for mutual induction is M. Now in this phenomenon when two or more than two coils are magnetically linked together by a the same magnetic flux then this property is known as mutual inductance.
The mutual inductance is the working principle of transformers, motors, generators and the electrical components which are interating with the magnetic field. Thus, in mutual inductance the electrical current flowing in one coil produces the voltage in the neighbouring coil. However, mutual inductance may have the stray inductance from the coil which interfere in the operation of neighbour coil by the phenomenon of electromagnetic induction.
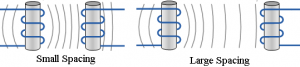
The amount of mutual inductance which links one coil to another is mainly dependant on the positions of the two coils. Consider a coil is positioned next to another coil at small distance, then the magnetic flux generated by the first coil interact with the coil turns of the second coil and induces large emf and hence produces the mutual inductance. On the other hand, if coils are separated from each other with larger distance then the induced magnetic flux from first coil into the second is lower, hence small emf is induced and hence mutual inductance is lower. Thus the effect of mutual inductance depends upon distance between the two coils. This phenomenon of mutual inductance is shown in figure below,
The mutual inductance between the two coils can be increased by putting them on a common soft iron core and by increasing the number of turns. When the coils are wound one on top of the other in the same iron coupling exist between them leads to smaller leakage flux. By assuming a perfect flux linkage between the two coils the mutual inductance is given by,
Where, µo is the permeability of free space (4.π.10-7), µr is the relative permeability of the soft iron core, N is in the number of coil turns, A is in the cross-sectional area in m2 and l is the coils length in meters.